Right Hand Rule in Physics
The right hand rule is a hand mnemonic used in physics to identify the direction of axes or parameters that point in three dimensions. Invented in the 19th century by British physicist John Ambrose Fleming for applications in electromagnetism, the right hand rule is most often used to determine the direction of a third parameter when the other two are known (magnetic field, current, magnetic force). There are a few variations of the right hand rule, which are explained in this section.
When a conductor, such as a copper wire, moves through a magnetic field (B), an electric current (I) is induced in the conductor. This phenomenon is known as Faraday's Law of Induction. If the conductor is moved inside the magnetic field, then there is a relationship between the directions of the conductor's motion (velocity), magnetic field and the induced current. We can use Fleming's right hand rule to investigate Faraday's Law of Induction, which is represented by the equation:
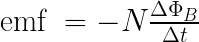
emf = induced emf (V or J/C)
N = number of turns of coil
Δ𝚽B = change in the magnetic flux (Tm2)
Δt = change in time (s)
Because the x, y and z axes are perpendicular to one another and form right angles, the right hand rule can be used to visualize their alignment in three-dimensional space. To use the right hand rule, begin by making an L-shape using your right thumb, pointer and middle finger. Then, move your middle finger inwards toward your palm, so that it is perpendicular to your pointer finger and thumb. Your hand should look similar to this:
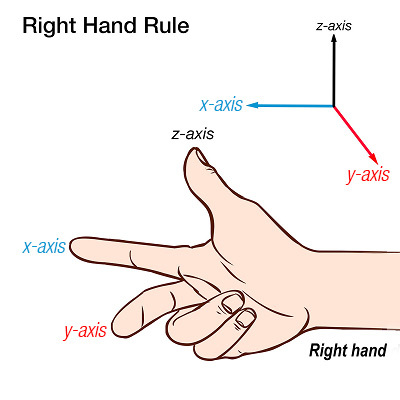
In the diagram above, the thumb aligns with the z axis, the index finger aligns with the x axis and the middle finger aligns with the y axis.
Teaching Tools
Wireless Smart Cart
One of the best ways to help students become confident using the right hand rule, is to perform a visual demonstration that helps them recognize and correct their misconceptions about orthogonal relationships and coordinate systems.
Right Hand Rule for Magnetism
Moving Charges
A charged particle is a particle with an electric charge. When a motionless charged particle exists in a magnetic field, it does not experience a magnetic force; however, as soon as the charged particle moves within a magnetic field, it experiences an induced magnetic force that displaces the particle from its original path. This phenomenon, also known as Lorentz force, is consistent with the rule that states, "magnetic fields do no work.” The equation used to determine the magnitude of the magnetic force acting on a charged particle (q) moving a magnetic field (B) with a velocity of v at an angle of θ is:
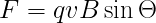
If the velocity of the charged particle is parallel to the magnetic field (or antiparallel), then there is no force because sin(θ) equals zero. When this occurs, the charged particle can maintain its straight line motion, even in the presence of a strong magnetic field.
The plane formed by the direction of the magnetic field and the charged particle’s velocity is at a right angle to the force. Because the force occurs at a right angle to the plane formed by the particle’s velocity and the magnetic field, we can use the right hand rule to determine their orientation.
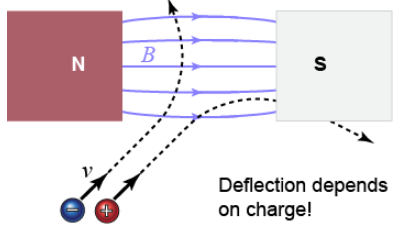
The right hand rule states that: to determine the direction of the magnetic force on a positive moving charge, point your right thumb in the direction of the velocity (v), your index finger in the direction of the magnetic field (B), and your middle finger will point in the direction of the the resulting magnetic force (F). Negative charges will be affected by a force in the opposite direction.
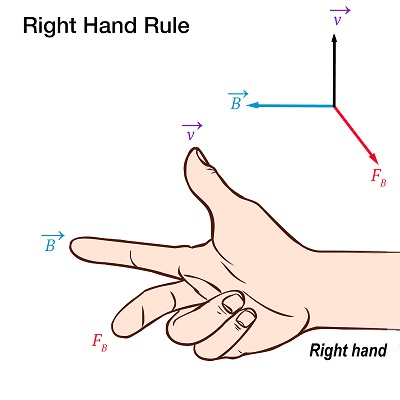
Current-Induced Magnetic Force: Current in a Straight Wire
A conventional current is composed of moving charges that are positive in nature. When a conventional current moves through a conducting wire, the wire is affected by a magnetic field that pushes it. We can use the right hand rule to identify the direction of the force acting on the current-carrying wire. In this model, your fingers point in the direction of the magnetic field, your thumb points in the direction of the conventional current running through the wire, and your palm indicates the direction that the wire is being pushed (force).
The magnetic force acting on a current-carrying wire is given by the equation:
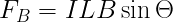
When the length of the wire and the magnetic field are at right angles to one another, then the equation becomes:
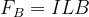
FB = magnetic force (N)
I = current (A)
L = length of wire (m)
B = magnetic field (T)
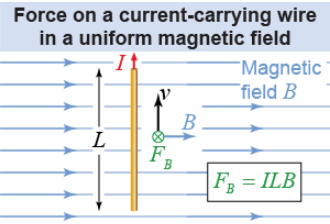
If we consider current flow as the movement of positive charge carriers (conventional current) in the above image, we notice that the conventional current is moving up the page. Since a conventional current is composed of positive charges, then the same current-carrying wire can also be described as having a current with negative charge carriers moving down the page. Although these currents are moving in opposite directions, a single magnetic force is observed acting on the wire. Therefore, the force occurs in the same direction whether we consider the flow of positive or negative charge carriers in the above image. Applying the right hand rule to the direction of the conventional current indicates the direction of the magnetic force to be pointed right. When we consider the flow of negative charge carriers in the above image, the right hand rule indicates the direction of the force to be left; however, the negative sign reverses the result, indicating that the direction of the magnetic force is indeed pointing right.
If we consider the flow of charges in two different wires, one with positive charges flowing up the page, and one with negative charges flowing up the page, then the direction of the magnetic forces will not be the same, because we are considering two different physical situations. In the first wire, the flow of positive charges up the page indicates that negative charges are flowing down the page. Using the right hand rule tells us that the magnetic force will point in the right direction. In the second wire, the negative charges are flowing up the page, which means the positive charges are flowing down the page. As a result, the right hand rule indicates that the magnetic force is pointing in the left direction.
Currents Induced by Magnetic Fields
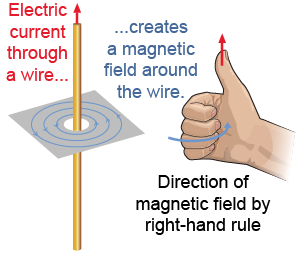
While a magnetic field can be induced by a current, a current can also be induced by a magnetic field. We can use the second right hand rule, sometimes called the right hand grip rule, to determine the direction of the magnetic field created by a current. To use the right hand grip rule, point your right thumb in the direction of the current's flow and curl your fingers. The direction of your fingers will mirror the curled direction of the induced magnetic field.
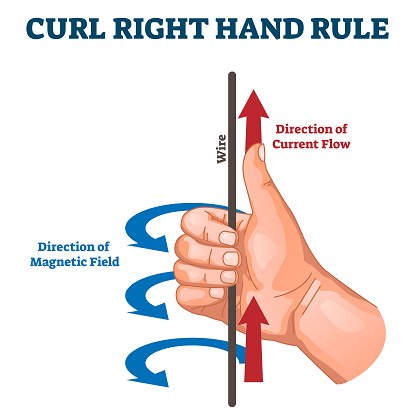
The right hand grip rule is especially useful for solving problems that consider a current-carrying wire or solenoid. In both situations, the right hand grip rule is applied to two applications of Ampere's circuital law, which relates the integrated magnetic field around a closed loop to the electric current passing through the plane of the closed loop.
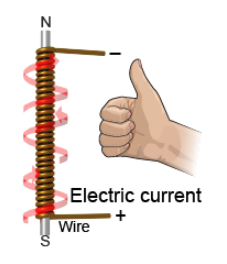
Rotational Direction: Solenoids
When an electric current passes through a solenoid, it creates a magnetic field. To use the right hand grip rule in a solenoid problem, point your fingers in the direction of the conventional current and wrap your fingers as if they were around the solenoid. Your thumb will point in the direction of the magnetic field lines inside the solenoid. Note that the magnetic field lines are in the opposite direction outside the solenoid. They wrap around from the inside to the outside of the solenoid.
Rotational Direction: Current-Carrying Wires
When an electric current passes through a straight wire, it induces a magnetic field. To apply the right hand grip rule, align your thumb with the direction of the conventional current (positive to negative) and your fingers will indicate the direction of the magnetic lines of flux.
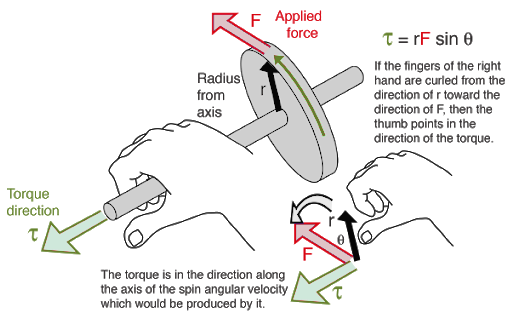
Right Hand Rule for Torque
Torque problems are often the most challenging topic for first year physics students. Luckily, there's a right hand rule application for torque as well. To use the right hand rule in torque problems, take your right hand and point it in the direction of the position vector (r or d), then turn your fingers in the direction of the force and your thumb will point toward the direction of the torque.
The equation for calculating the magnitude of a torque vector for a torque produced by a given force is:

When the angle between the force vector and the moment arm is a right angle, the sine term becomes 1 and the equation becomes:
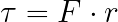
F = force (N)
𝜏 = torque (Nm)
r = distance from center to line of action (m)
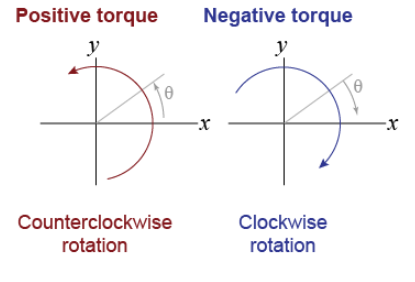
Positive and Negative Torques
Torques that occur in a counter clockwise direction are positive torques. Alternatively, torques that occur in the clockwise direction are negative torques. So what happens if your hand points in or out of the paper? Torques that face out from the paper should be analyzed as positive torques, while torques that face inwards should be analyzed as negative torques.
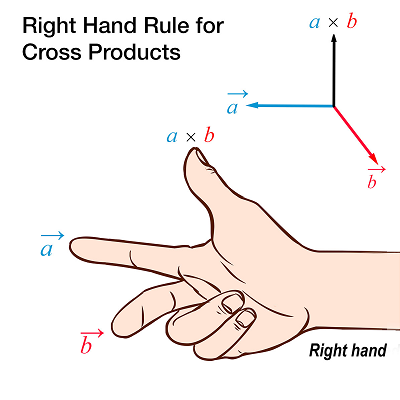
Right Hand Rule for a Cross Product
A cross product, or vector product, is created when an ordered operation is performed on two vectors, a and b. The cross product of vectors a and b, is perpendicular to both a and b and is normal to the plane that contains it. Since there are two possible directions for a cross product, the right hand rule should be used to determine the direction of the cross product vector.
For example, the cross product of vectors a and b can be represented using the equation:
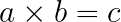
(pronounced “a cross b”)
To apply the right hand rule to cross products, align your fingers and thumb at right angles. Then, point your index finger in the direction of vector a and your middle finger in the direction of vector b. Your right thumb will point in the direction of the vector product, a x b (vector c).
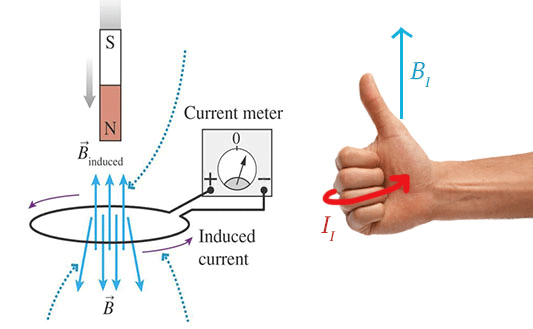
Right Hand Rule for Lenz's Law
Lenz's law of electromagnetic induction is another topic that often seems counterintuitive, because it requires understanding how magnetism and electric fields interact in various situations. Lenz's law states that the direction of the current induced in a closed conducting loop by a changing magnetic field (Faraday's Law) is such that the secondary magnetic field created by the induced current opposes the initial change in the magnetic field that produced it. So what does this mean? Let's break it down.
When the magnetic flux through a closed loop conductor changes, it induces a current within the loop. The induced current creates a secondary magnetic field that opposes the original change in flux that initiated the induced current. The strength of the magnetic field passing through a wire coil determines the magnetic flux. Magnetic flux depends on the strength of the field, the area of the coil, and the relative orientation between the field and the coil, as shown in the following equation.
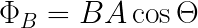
𝚽B = magnetic flux (Tm2)
B = magnetic field (T)
Θ = angle between field and normal (deg)
A = area of loop (m2)
To understand how Lenz's Law will affect this system, we need to first determine whether the initial magnetic field is increasing or decreasing in strength. As the magnetic north pole gets closer to the loop, it causes the existing magnetic field to increase. Since the magnetic field is increasing, the induced current and resulting induced magnetic field will oppose the original magnetic field by reducing it. This means that the primary and secondary magnetic fields will occur in opposite directions. When the existing magnetic field is decreasing, the induced current and resulting induced magnetic field will oppose the original, decreasing magnetic field by reinforcing it. Thus, the induced magnetic field will have the same direction as the original magnetic field.
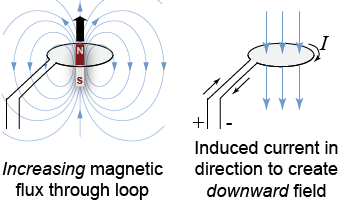
To apply the right hand rule to Lenz's Law, first determine whether the magnetic field through the loop is increasing or decreasing. Recall that magnets produce magnetic field lines that move out from the magnetic north pole and in toward the magnetic south pole. If the magnetic field is increasing, then the direction of the induced magnetic field vector will be in the opposite direction. If the magnetic field in the loop is decreasing, then the induced magnetic field vector will occur in the same direction to replace the original field's decrease. Next, align your thumb in the direction of the induced magnetic field and curl your fingers. Your fingers will point in the direction of the induced current.